310801: CF1889F. Doremy's Average Tree
Description
Doremy has a rooted tree of size $n$ whose root is vertex $r$. Initially there is a number $w_i$ written on vertex $i$. Doremy can use her power to perform this operation at most $k$ times:
- Choose a vertex $x$ ($1 \leq x \leq n$).
- Let $s = \frac{1}{|T|}\sum_{i \in T} w_i$ where $T$ is the set of all vertices in $x$'s subtree.
- For all $i \in T$, assign $w_i := s$.
Doremy wants to know what is the lexicographically smallest$^\dagger$ array $w$ after performing all the operations. Can you help her?
If there are multiple answers, you may output any one.
$^\dagger$ For arrays $a$ and $b$ both of length $n$, $a$ is lexicographically smaller than $b$ if and only if there exist an index $i$ ($1 \leq i \le n$) such that $a_i < b_i$ and for all indices $j$ such that $j<i$, $a_j=b_j$ is satisfied.
InputThe input consists of multiple test cases. The first line contains a single integer $t$ ($1\le t\le 10^4$) — the number of test cases. The description of the test cases follows.
The first line contains three integers $n$, $r$, $k$ ($2 \le n \le 5000$, $1 \le r \le n$, $0 \le k \le \min(500,n)$).
The second line contains $n$ integers $w_1,w_2,\ldots,w_n$ ($1 \le w_i \le 10^6$).
Each of the next $n-1$ lines contains two integers $u_i$, $v_i$ ($1 \leq u_i, v_i \leq n$), representing an edge between $u_i$ and $v_i$.
It is guaranteed that the given edges form a tree.
It is guaranteed that the sum of $n$ does not exceed $50\,000$.
OutputFor each test case, In the first line, output a single integer $cnt$ ($0 \le cnt \le k$) — the number of operations you perform.
Then, in the second line output $cnt$ integers $p_1,p_2,\ldots,p_{cnt}$ — $x$ is chosen to be $p_i$ for $i$-th operation.
If there are multiple answers, you may output any one.
ExampleInput4 6 1 1 1 9 2 6 1 8 1 2 1 3 2 4 3 6 3 5 7 7 2 3 1 3 3 1 1 2 7 1 7 2 7 4 1 5 2 3 4 6 6 5 1 3 1 3 1 1 3 5 3 5 1 5 6 3 4 1 2 3 2 1 1000000 999999 999997 2 1 1 3Output
1 2 2 1 4 1 5 1 1Note
In the first test case:
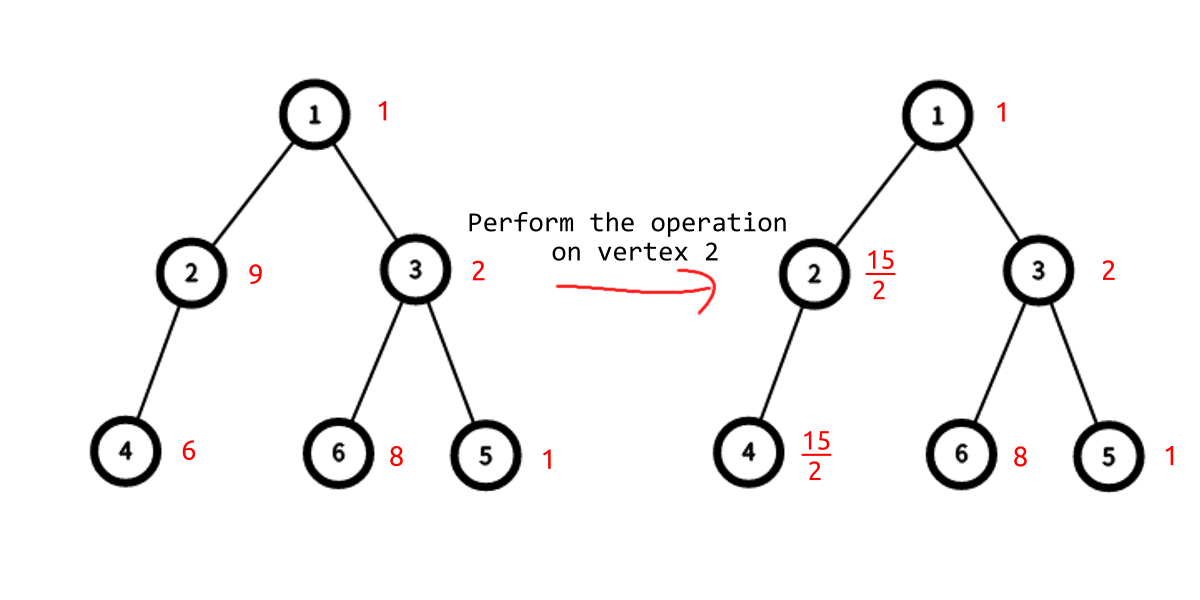
At first $w=[1,9,2,6,1,8]$. You can choose some vertex $x$ to perform at most one operation.
- If $x=1$, $w=[\frac{9}{2},\frac{9}{2},\frac{9}{2},\frac{9}{2},\frac{9}{2},\frac{9}{2}]$.
- If $x=2$, $w=[1,\frac{15}{2},2,\frac{15}{2},1,8]$.
- If $x=3$, $w=[1,9,\frac{11}{3},6,\frac{11}{3},\frac{11}{3}]$.
- If $x \in \{4, 5, 6\}$, $w=[1,9,2,6,1,8]$.
- If you don't perform any operation, $w=[1,9,2,6,1,8]$.
$w$ is lexicographically smallest when $x=2$.
Output
Doremy有一棵大小为n的以r为根的树。最初,顶点i上写有数字w_i。Doremy可以使用她的力量执行以下操作最多k次:
1. 选择一个顶点x(1≤x≤n)。
2. 让s=1/|T| * ∑(i属于T) w_i,其中T是x子树中所有顶点的集合。
3. 对于所有i属于T,赋值w_i:=s。
Doremy想知道执行所有操作后,w数组字典序最小的是什么。你能帮她吗?
如果有多个答案,你可以输出任何一个。
**输入数据格式:**
输入由多个测试用例组成。第一行包含一个整数t(1≤t≤10^4)——测试用例的数量。接下来是测试用例的描述。
每个测试用例的第一行包含三个整数n、r、k(2≤n≤5000,1≤r≤n,0≤k≤min(500,n))。
第二行包含n个整数w_1,w_2,…,w_n(1≤w_i≤10^6)。
接下来n-1行,每行包含两个整数u_i、v_i(1≤u_i,v_i≤n),表示u_i和v_i之间的边。
保证给定的边构成一棵树。
保证n的总和不超过50,000。
**输出数据格式:**
对于每个测试用例,在第一行输出一个整数cnt(0≤cnt≤k)——你执行的操作数。
然后在第二行输出cnt个整数p_1,p_2,…,p_cnt——第i次操作选择的x是p_i。
如果有多个答案,你可以输出任何一个。
**公式(LaTeX格式):**
- 平均值计算公式:$$
s = \frac{1}{|T|} \sum_{i \in T} w_i
$$
**注意:**
- 字典序最小:对于长度为n的数组a和b,如果存在一个索引i(1≤i≤n),使得a_i < b_i,并且对于所有索引j(j < i),a_j = b_j,则a在字典序上小于b。**题目大意:** Doremy有一棵大小为n的以r为根的树。最初,顶点i上写有数字w_i。Doremy可以使用她的力量执行以下操作最多k次: 1. 选择一个顶点x(1≤x≤n)。 2. 让s=1/|T| * ∑(i属于T) w_i,其中T是x子树中所有顶点的集合。 3. 对于所有i属于T,赋值w_i:=s。 Doremy想知道执行所有操作后,w数组字典序最小的是什么。你能帮她吗? 如果有多个答案,你可以输出任何一个。 **输入数据格式:** 输入由多个测试用例组成。第一行包含一个整数t(1≤t≤10^4)——测试用例的数量。接下来是测试用例的描述。 每个测试用例的第一行包含三个整数n、r、k(2≤n≤5000,1≤r≤n,0≤k≤min(500,n))。 第二行包含n个整数w_1,w_2,…,w_n(1≤w_i≤10^6)。 接下来n-1行,每行包含两个整数u_i、v_i(1≤u_i,v_i≤n),表示u_i和v_i之间的边。 保证给定的边构成一棵树。 保证n的总和不超过50,000。 **输出数据格式:** 对于每个测试用例,在第一行输出一个整数cnt(0≤cnt≤k)——你执行的操作数。 然后在第二行输出cnt个整数p_1,p_2,…,p_cnt——第i次操作选择的x是p_i。 如果有多个答案,你可以输出任何一个。 **公式(LaTeX格式):** - 平均值计算公式:$$ s = \frac{1}{|T|} \sum_{i \in T} w_i $$ **注意:** - 字典序最小:对于长度为n的数组a和b,如果存在一个索引i(1≤i≤n),使得a_i < b_i,并且对于所有索引j(j < i),a_j = b_j,则a在字典序上小于b。